Problem of the Fortnight
This page houses the MGSA “Problem of the Fortnight” puzzles for the Spring of 2021. This is open to graduate students, professors, undergraduate students, and anyone else who wants to participate. At the end of the semester we will do a raffle drawing for prizes, with each puzzle being an opportunity to earn more entries in the raffle:
Submitting a solution earns you one entry.
If your solution is correct you earn an additional entry.
If your solution is chosen as the “best” (or prettiest) solution you will earn two more entries for a total of four entries!
To submit a solution click here: https://forms.gle/aqenP9oZEwshas4XA
Problem 5: A Strange Game (4/12/21-4/26/21)
A square with sides 75 cm long is drawn. Mr. Wuf and Mrs. Wuf take turns drawing circles with radius between 1 cm and 4 cm inside the square. The circles are not allowed to intersect or be drawn inside another circle. The first player who cannot draw a circle in the remaining space inside the square loses. Mrs. Wuf has the honor of drawing the first circle. Does either player have a winning strategy? If so, describe it.
Correct Solutions were submitted by Dylan Bates and Jordan Almeter. Dylan’s solution can be seen here
Problem 4: Strawberry Ice Cream (3/29/21-4/11/21)
A mathematician walks into a bar on Main Street, orders a drink, and starts chatting with the bartender. After a while, she learns that the bartender has three children. “How old are your children?” she asks. “Well,” replies the bartender, “the product of their ages is 72.” The mathematician waits for a second and then says, “Ok, can I get some more information?” “All right,” continues the bartender, “if you go outside and look at the building number posted over the door to the bar, you’ll see the sum of their ages.” The mathematician steps outside, and after a few moments she reenters and declares, “That is still not enough information.” The bartender smiles and says, “My youngest just loves strawberry ice cream.” The mathematician is finally satisfied as she now knows the children’s ages.
How old are the three children?
Correct Solutions were submitted by: Yiwei Zhang, Clayton Hansen, Sean Thompson, Walker Powell, Christopher Leonard, Tiancheng Xue, Marco Hamins-Puertolas, Dylan Bates, Shane Newman, Tim Ablondi, Steven Gilmore, and Alex Harp. Steven Gilmore’s solution can be seen here.
Problem 3: Forgotten Boarding Pass (3/15/21-3/28/21)
100 people board a fully booked aircraft and each person has a seat assigned to them. Unfortunately, the first person in line somehow loses their boarding pass and therefore doesn’t know which seat is assigned to them. They decide to take a random seat and hope for the best. Each successive passenger takes their assigned seat, if it is empty; otherwise they take a random vacant seat. What is the probability that the last passenger finds their assigned seat unoccupied?
Correct Solutions were submitted by: Dylan Bates, Ezra Nance, Sean Thompson, and Alex Harp. Alex Harp’s solution can be seen here.
Problem 2: A Tale of Two Circles (3/1/21-3/14/21)
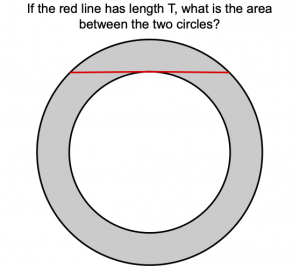
Correct Solutions were submitted by: Eric Geiger, Dylan Bates, Nikki Xu, Angela Vichitbandha, Kuangying Li, Sean Thompson, Jiewen Sheng, Ezra Nance, Christopher Leonard, Prerona Dutta, Jordan Almeter, Tim Reid, Walker Powell, Steven Gilmore, and Alex Harp. Here is Ezra Nance’s solution.
Problem 1: A “Counting” Problem (2/15/21-3/1/21)
In the sequence of sentences below, fill in each blank with the numbers 0, 1, 2, 3, 4, 5, 6, 7, 8, 9 in such a way so that all sentences are true at the same time. Every number must be used at least once.
- The number __ appears __ times.
- The number __ appears __ times.
- The number __ appears __ times.
- The number __ appears __ times.
- The number __ appears __ times.
- The number __ appears __ times.
- The number __ appears __ times.
- The number __ appears __ times.
- The number __ appears __ times.
- The number __ appears __ times.
For a bonus entry to the prize raffle at the end of the semester: Other than permuting the order of the sentences, are there any other solutions?
Correct Solutions were submitted by: Zev Woodstock, Evan North, Eric Geiger, Adam Pickarski, Marco Hamins-Puertolas, Ezra Nance, Sean Thompson, Jordan Almeter, Dylan Bates, Clayton Hansen, and Hassan Hatam. Here is Jordan Almeter’s solution.
